Number Systems

Number Types

The number system holds significance in terms of writing and expressing code to a computer, typically in a programming language. Note that we (as humans) do not use hex or binary numbers that much outside of the computer science domain. For example, we don't walk into a supermarket and read prices in binary such as $0x10 :)

Often times in programming, we need to express numbers more quickly, and we might say int x = 0x10000000 to quickly indicate 32-bit value with bit31 set to 1. Notation x = 0x10000000 is easier than writing x = 268435456 which would be more cryptic for a programmer to realize the significance of because the reader of the programming code will not be able to quickly realize that it is specifically setting bit31 to value of 1.

Decimal

Typical numbers we are familiar with are decimals which are technically "base 10" numbers. So an ordinary number that we may be aware of such as 123 can be written as 123₁₀.

The number 123 could also be written as:

1*102 +2*101 +3*100 which is equal to 100 + 20 + 3 =12310

Binary

Binary numbers are always 1s and 0s only. Similar to decimal numbers, binary numbers increase in powers of 2, rather than powers of 10. Binary numbers are written by with the "0b" notation, such as 0b1100

For example, binary 101 or 0b101 can be written as:

1*22 +0*21 +1*20 which is equal to 4 + 0 + 1 = 510

Hex

One digit of a hex number can count from 0-15, but since we have to represent the hex number using a single character, the numbers 0-9 are usual numbers, and the numbers 10-15 are represented by A, B, C, D, E, F

Where decimal is a power of 10, and binary is power of 2, hex numbers are powers of 16. Hex numbers are written with the "0x" notation, such as 0x10.

For example, hex 0x12 can be written as:

1*161 +2*160 which is equal to 16 + 2 = 1810

As another example, hex 0xC5 can be written as:

12*161 +5*160 which is equal to 192 + 5 = 19710

Exercises

Decimal to Binary

Decimal (base 10) numbers can be converted in a couple of different ways as described here. One of the methods is to continue dividing by 2 and note down the remainder as described in the image below. The article above also describes a potentially faster method of conversion so be sure to read it!

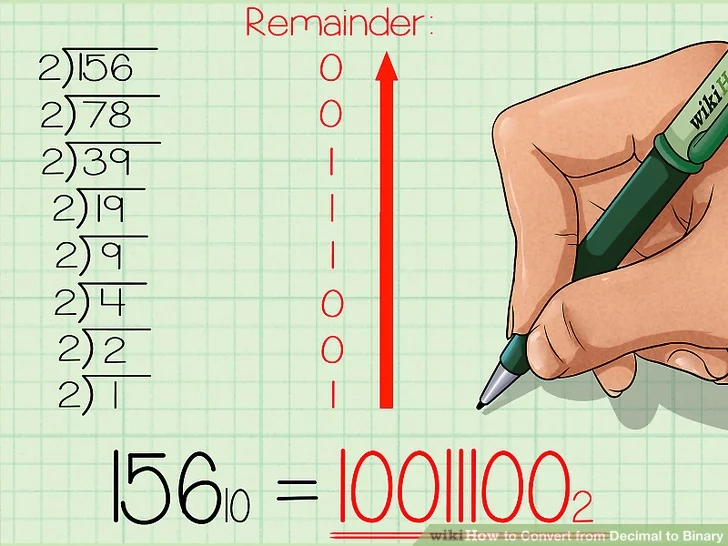
Please try converting the following to binary:

1. 125
2. 255
3. 500

Decimal to Hex

Decimal to hex is similar to Decimal to Binary except that we are dealing with powers of 16 rather than powers of 2.

My favorite method of conversion from decimal to hex is to first convert the number to binary. For example, let's start with a large number such as 23912. We can use the Decimal to Binary method to convert this first to binary:

• 2391210
• 0b101110101101000
• Split it up to nibbles:
  • 0b101 1101 0110 1000
• Then use the lookup table listed in Hex to Binary:
  • 0x5D68

Please try converting the following to hex:

1. 125
2. 255
3. 500

Hex to Binary

The following table can be utilized to convert hex to binary very instantly:

First row is HEX, and the second row is binary. For whatever hex number we wish to convert, we simply locate its equivalent in binary. For instance, if we wish to convert 0x5 to binary, it is 0b0101, and 0xA5 would be 0b1010.0101 as you can convert one "nibble" (4-bits) at a time.

Let's take another example to convert 0x1BF to binary; simply break it down by "nibbles":

• 0x1 --> 0b0001
• 0xB --> 0b1011
• 0xF --> 0x1111
• Answer: 0b0001 1011 1111 

Please try converting the following to binary:

1. 0x55
2. 0x125
3. 0x40000000

Hex to Decimal

For Hex to Binary, we used a lookup table as a "cheat code" :). For Hex to decimal, it would be easier to re-write the numbers as powers of 16. For example, to convert 0x1BF to decimal, we can break it down to:

• 0x1 --> 1 * 162 --> 256
• 0xB --> 11 * 161 --> 176
• 0xF --> 15 * 160 --> 15
• 256+176+15 = 447

Please try converting the following to decimal:

1. 0x55
2. 0x125
3. 0x40000000